List of illustrations page x List of tables xii Preface xiii List of abbreviations xvi 1 Introduction 1 1.1 Interference modeling and performance evaluation 2 1.1.1 Mathematical modeling 2 1.1.2 Experimental modeling 3 1.1.3 Simulation modeling 3 1.2 Interference avoidance and coexistence strategies 4 1.2.1 Industry led activities 5. 4.1 General 4.2 Experimental 4.3 Results and Discussion I 4.3.1 4.3.2 4.3.3 5. Conclusions Reproducibility Molecular Weight Cal ibration Accounting for Imperfect Resolution 4.3.3.1 4.3.3.2 Evaluation of Existing Methods Development of New Methods 4.3.3.2.1 Prel iminary Investigation 4.3.3.2.2 The Method of. . theoretical probability (p. 782). experimental probability (p. 782) Key Vocabulary The United States Senate forms committees to focus on different issues. These committees are made up of senators from various states and political parties. There are many ways these committees could be formed.You will.
- 4.1 Experimental And Theoretical Probabilitymr. Mac's Page Examples
- 4.1 Experimental And Theoretical Probabilitymr. Mac's Page Sheet
- 4.1 Experimental And Theoretical Probabilitymr. Mac's Page Key
- 4.1 Experimental And Theoretical Probabilitymr. Mac's Page Example
Probability
Probability is a measure of the likelihood that an event will happen.
When dealing with probability, the outcomes of a process are thepossible results. For example, when a die is rolled, the possibleoutcomes are 1, 2, 3, 4, 5, and 6. In mathematicallanguage, an event is a set of outcomes, which describe whatoutcomes correspond to the 'event' happening. For instance, 'rolling aneven number' is an event that corresponds to the set of outcomes{2, 4, 6}.The probability of an event, like rolling an even number, is thenumber of outcomes that constitute the event divided by the totalnumber of possible outcomes. We call the outcomes in an event its'favorable outcomes'.
Calculate the theoretical & experimental probabilities of rolling a 4 in simplest form. Experimental Probability Anthony is going to spin the 6-sectioned spinner below to see if he really will get the color Yellow 1/6 of the time. 4.1 VMD The VMD source code and binary distributions can be obtained after registering at the VMD web page. Download the appropriate distribution file with your web browser. Windows binary distributions are self extracting, so once the distribu-tion file is downloaded, proceed to the installation directions. For source distri.
If a die is rolled once, determine the probability of rolling a4: Rolling a 4 is an event with 1 favorable outcome (aroll of 4) and the total number of possible outcomes is 6 (a rollof
 1, 2, 3, 4, 5, or 6). Thus, the probability of rollinga 4 is .
1, 2, 3, 4, 5, or 6). Thus, the probability of rollinga 4 is .If a die is rolled once, determine the probability of rollingat least a 4: Rolling at least 4 is an event with 3favorable outcomes (a roll of 4, 5, or 6) and the total numberof possible outcomes is again
4.1 Experimental And Theoretical Probabilitymr. Mac's Page Examples
64.1 Experimental And Theoretical Probabilitymr. Mac's Page Sheet
. Thus, the probability of rolling atleast a4.1 Experimental And Theoretical Probabilitymr. Mac's Page Key
4 is = .Here are two more examples:
If a coin is flipped twice, determine the probability that it will land heads both times:
Favorable outcomes: 1 -- HH
Possible outcomes: 4 -- HH, HT, TH, TT
Thus, the probability that the coin will land heads both times is .
If Dan grabs one sock from a drawer containing 3 white socks, 4 blue socks, and 5 yellow socks, what is the probability that he will grab a white sock?
Favorable outcomes: 3 (3 white socks)
Possible outcomes: 12 (3 white socks + 4 blue socks + 5 yellow socks)
Thus, the probability that Dan will grab a white sock is = .
Though probabilities are calculated as fractions, they can beconverted to decimals or percents--the Fractions SparkNotein Pre-Algebra explains how to convert fractions to decimalsand the SparkNote on Percents describes how toconvert them to percents.
Boundaries on Probability
If all outcomes are favorable for a certain event, its probability is 1. For example, the probability of rolling a 6 or lower on one die is = 1.
4.1 Experimental And Theoretical Probabilitymr. Mac's Page Example
If none of the possible outcomes are favorable for a certainevent (a favorable outcome is impossible), the probability is 0.For example, the probability of rolling a 7 on one die is = 0.
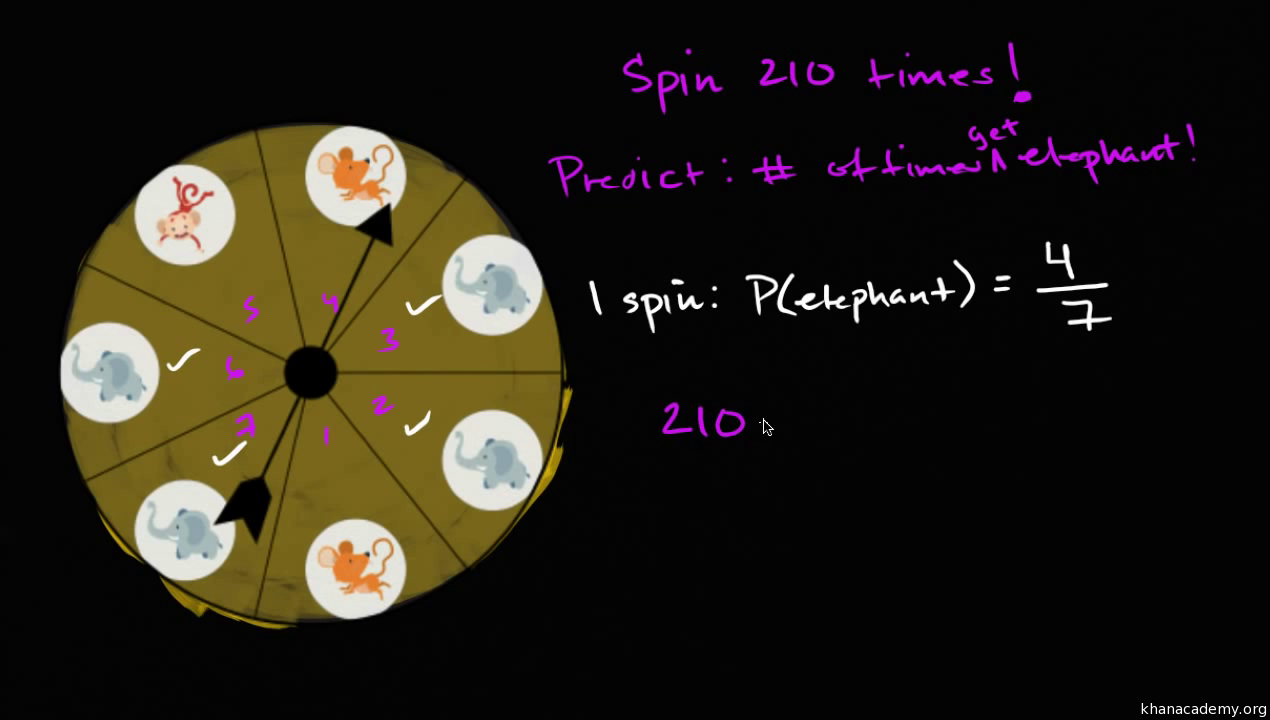
b. Joan picks a random counter out of a bag 7 times. What is the experimental a. What is the theoretical probability of pickinga yellow counter in simplest form? Sean is doing an experiment to see how many tails Trial 1 4 6 Heads Tails Tails b. What is the experimental (in simplest form) a. What is the theoretical How many times would he expect to get the color red if he did 30 spins? 18 times 60 times He gets the color red 6 times. What is the experimental probability of getting blue? Green Yellow Blue Diana completes an experiment of spinning athree-colored spinner 15 times. She expected to get aprobability of 1/3 for each color, but her numberswere really off. She got 0% probability for red, 60% for green, and 40% for blue. How could she get her experimental probability closer to her theoretical probability? She could spin a different spinner. She needs to spin fewer times. the theoretical probability is always less than the experimental probability the more times the cube is rolled, the closer the experimental probability gets to the theoretical probability the theoretical probability is always greater than the experimental probability. Which of the following statements is TRUE? When rolling a number cube... Mr. Whatley rolled a fair number cube 50 times. The number 4 came up 10 times. Calculate the theoretical& experimentalprobabilities of rolling a 4 in simplest form. Anthony is going to spin the 6-sectioned spinner below to see if he really will get the colorYellow 1/6 of the time. How many times should he spin the spinner for the outcome of his experiment to best match his theory? 6 spins 1 spin |